Statistics 2 - Quadratic Regression Model Example

Quadratic Regression Model Example

baseball

Data: The table at the right shows the horizontal distance (in feet) traveled by a baseball hit at various angles. The initial speed of the ball at the bat is constant. Batter up!!!

Angle (degrees)	Distance (feet)
10°	115.6
15°	157.2
20°	189.2
24°	220.8
30°	253.8
34°	269.2
40°	284.8
45°	285.0
48°	277.4
50°	269.2
58°	244.2
60°	231.4
64°	180.4

Task: Express answers to 3 decimal places unless told otherwise.
	a.)	Prepare a scatter plot of the data.
	b.)	Determine a quadratic regression model equation to represent this data amd graph the new equation.
	c.)	Decide whether the new equation is a "good fit" to represent this data.
	d.)	Extrapolate data: What distance will correspond to an angle of a 5°?
	e.)	Interpolate data: What angle(s) will correspond to a distance of 270 feet, to the nearest degree?
	f.)	The first baseman is positioned 100 feet from home plate and the right fielder is positioned 180 feet from home plate. The batter wants to hit the ball half way between these players. What angle, to the nearest degree, should be used to accomplish this hit? Round to 3 decimal places.
	g.)	The left field fence is 280 feet from home plate. At what angle, or angles, to the nearest degree, will the ball be hit past the left field fence?

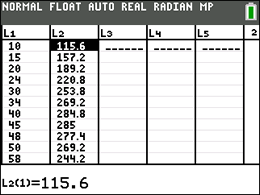
Step 1. Enter the data into the lists. For basic entry of data, see Basic Commands.
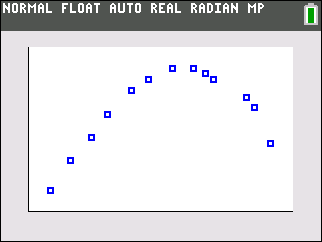
Step 2. Create a scatter plot of the data. Go to STATPLOT (2nd Y=) and choose the first plot. Turn the plot ON, set the icon to Scatter Plot (the first one), set Xlist to L1 and Ylist to L2 (assuming that is where you stored the data), and select a Mark of your choice. ZoomStat.	(answer to part a)
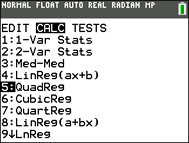
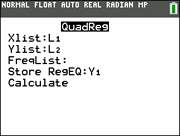
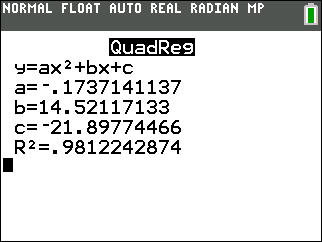
Step 3. Choose the Quadratic Regression Model. Press STAT, arrow right to CALC, and arrow down to 5: QuadReg. Hit ENTER. When the template for QuadReg appears on the home screen, type the parameters L1, L2, Y1. The Y1 will put the equation into Y= for you. (Y₁ comes from VARS → YVARS, #Function, Y₁or from ALPHA F4)	The quadratic regression equation is y = -0.173x² + 14.521x - 21.898 (answer to part b)
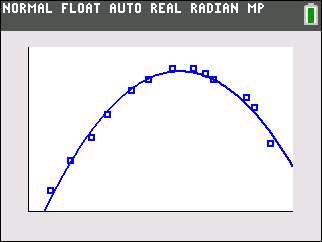
Step 4. Graph the Quadratic Regression Equation from Y1.	(answer to part b)
Step 5. Is this model a "good fit"? The coefficient of determination, R², is .9812242874 which means that 98% of the total variation in y can be explained by the relationship between x and y. Yes, it is a very "good fit". (answer to part c)
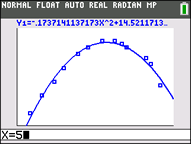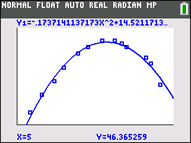
Step 6. Extrapolate: (beyond the data set) Get the answer from the graph of the regression equation. Check the WINDOW to be sure that 5º falls within the domain (it does).) Hit TRACE. Arrow up so the regression equation will show at the top of the screen. Type 5 and hit ENTER. (answer to part d -- At 5°, the distance will be 46.365 feet.)	Step 7. Interpolate: (within the data set) From TABLE, find the value in L2 closest to 270. Adjust the TBLSET to narrow in on the answer. 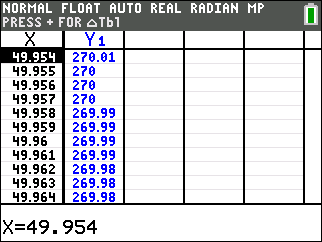(answer to part e -- angle will be 50° or 34º)
Step 8. The first baseman is positioned 100 feet from home plate and the right fielder is positioned 180 feet from home plate. The batter wants to hit the ball half way between these players. What angle, to the nearest degree, should be used to accomplish this hit?	Half way between 100 feet away and 180 feet away is 140 feet. Find the angle within the scatter plot domain that corresponds with 140 feet. 13 degrees (answer to part f).
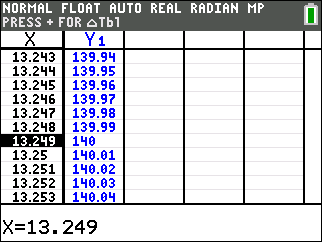
Step 9. The left field fence is 280 feet from home plate. At what angle, or angles, will the ball be hit past the left field fence?	Use the TABLE to find values greater than 280. Since the graph is parabolic, you are looking for two locations. (answer to part g) any angle between 39° and 44°.

Finding Your Way Around TABLE of CONTENTS